We note that the feed is in stoichiometric mix, so we are free to select either reactant as the reference species. Letting A be the reference we have

We calculate the following stoichiometric parameters:

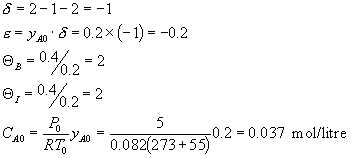
The concentrations of the various species, expressed as functions of X, are then

1. The concentrations of the various species at X=0.6 are then



2. In the absence of pressure drop we have the following

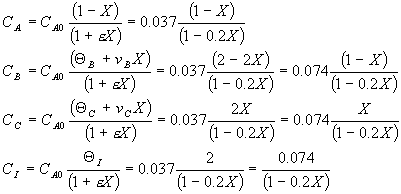
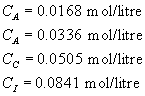
Design Equation



We note that and adjust k accordingly (i.e. k =0.037).

Rate Expression (in terms of species A)



 

Combining



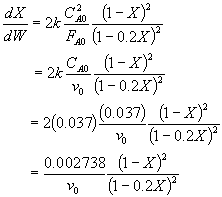
Note that the volumetric flow rate (or equivalently the molar flow

rate) is unspecified. We can carry this variable through and rearrange to give



This equation can be integrated directly (from table) to give



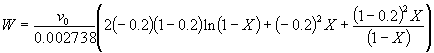
We can then solve for W at X=0.1.

(If you assumed a value for either F_A0 or v₀ or expressed W in terms of F_A0,

that's fine too).

 

3. With pressure drop, the concentrations are now functions of the pressure ratio:

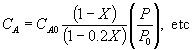
The new differential form of the design equation is then

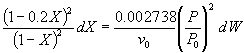
We need the Ergun equation as well. Since , we have

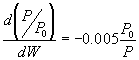
Integrating gives

We then substitute this into our differential equation to give

which can be integrated to give

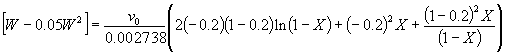
If you assumed a value for one of the flows here to yield a numerical value, that's

fine.